∫1 x^2 2x 3dx-搜答案-神马作文网

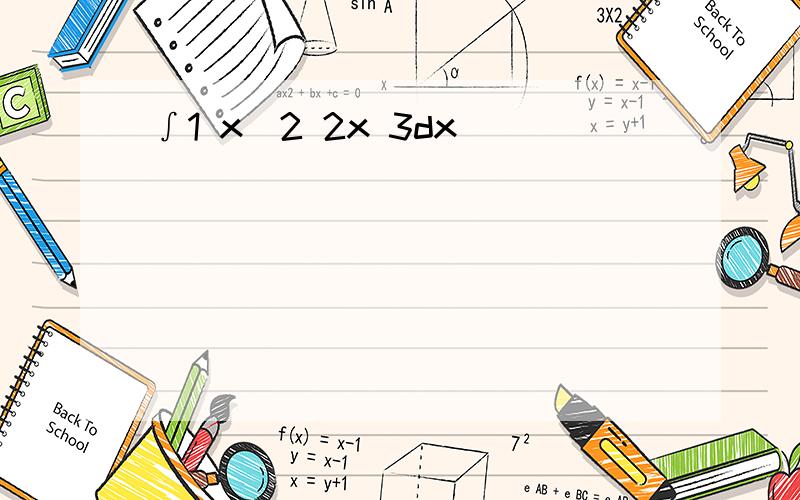
令t=x*sqrt(x);原式则=2/3*∫sqrt[1/(1-t)]dt＝-4/3sqrt(1-t)+C=-4/3*sqrt[1-x*sqrt(x)]+C

∫(X^2+X+1/X)dx=x^3/3+x^2/2+lnx+C

答案见附图

①=[(x+4x+8)+2x][(x+4x+8)+x]=(x+6x+8)(x+5x+8)=(x+2)(x+4)(x+5x+8)②=(2x-3x+1)-22x+33x-1=(2x-3x+1)-22x+3

再问：详细点再问：这变的好突然再答：哦，看来是这不懂啊，把分母拆开，二次项那个分子设为AX+B，一次项那个设为C，两个分式相加分子等于x，就可以把ABC解出来了再问：好深奥的样子。。。我问问数学课代表

当x=0时,f(x)不连续,故f(x)的原函数分成两部分：x>0,∫f(x)dx=∫x㏑(1+x^2)dx=(1/2)∫㏑(1+x^2)d(x^2)=(1/2)ln|ln(1+x^2)|+C1x

∫1+2x/x(1+x)*dx求解

你好∫(1+2x)/x(1+x)*dx=∫(1+x+x)/x(1+x)*dx=∫[1/(1+x)+1/x]*dx=ln(x+1)+lnx+C很高兴为您解答,祝你学习进步!有不明白的可以追问!如果有其他

设u=ln(1+x)-lnx.∫[ln(1+x)-lnx]/x(1+x)dx=-∫udu=-1/2u²+C=-1/2[ln(1+x)-lnx]²+C

∫(x^3+1)/(x(1-x^3))dx

(1+x³)/[x(1-x³)]=(1+x³)/[x(1-x)(1+x+x²)]令(1+x³)/[x(1-x)(1+x+x²)]=A/(1+

∫f'(x)dx/1+f^2(x)=∫df(x)/[1+f^2(x)]=arctanf(x)+c=arctan(e^x/x)+c

/>(4X+2)/X+【4(X-5)-2】/(X-5)=【(X-4)-2】/(X-4)+【7(X+1)+2】/(X+1)4+2/X+4-2/(X-5)=1-2/(X-4)+7+2/(X+1)8+2/X

∫dx/x(1+x)

1/x(x+1)=1/x-1/(x+1)所以原式=∫[(ln(x+1)-lnx]*[1/x-1/(x+1)]dx=∫[(ln(x+1)-lnx]d[lnx-(ln(x+1)]=-∫[lnx-ln(x+

其实1/[x(x+1)]=(1/x)-1/(1+x)只不过是换了一种表达方式和位置而已

(1)∫[-1,0]x³dx=x⁴/4|[-1,0]=1/4表示函数y=x³与x=-1x=0所围成的图形的面积(2)f(x)=x³∵f(-x)=-x³

∵(x^4-4x^2+5x-15)/[(x^2+1)(x-2)]=[(x^4+x²-5x²-5)+(5x-10)]/[(x²+1)(x-2)]=[x²(x&su

∫x*√[(1-x)/(1+x)]dx=∫[x(1-x)/√(1-x^2)]dxletx=sinydy=cosydy∫[x(1-x)/√(1-x^2)]dx=∫siny(1-siny)dy=∫[sin

[(x^3-2x^2+x+1)/(x^4+5x^2+4)]=1/(x^2+1)+(x-3)/(x^2+4).原式=∫1/(x^2+1)dx+∫(x-3)/(x^2+4)dx=arctanx+(1/2)

e^x-e^-x是奇函数x(x+x^2011)(e^x-e^-x)还是奇函数奇函数在-a到a的积分为0再问：我也觉得是0，不过答案是4/e再答：那你抄错题了若是∫[-1,1]x(1+x^2011)(e

再问：+c再答：对